Surface Simplification Using Intrinsic Error Metrics
| Hsueh-Ti Derek Liu | Mark Gillespie | Benjamin Chislett | Nicholas Sharp | Alec Jacobson | Keenan Crane |
ACM Trans. Graph. (2023)
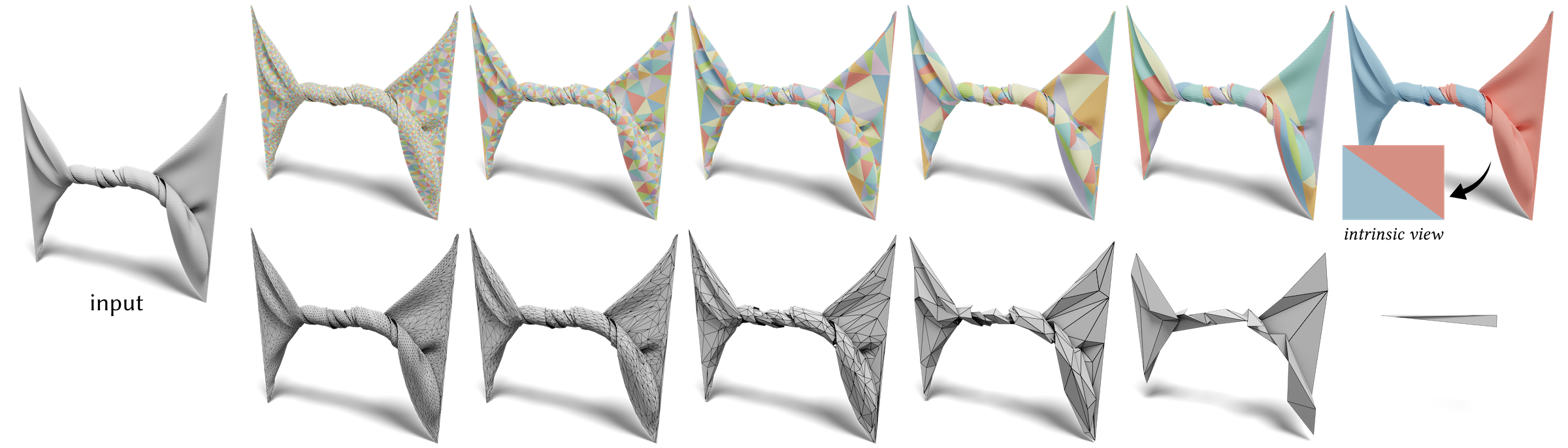
This paper describes a method for fast simplification of surface meshes. Whereas past methods focus on visual appearance, our goal is to solve equations on the surface. Hence, rather than approximate the extrinsic geometry, we construct a coarse intrinsic triangulation of the input domain. In the spirit of the quadric error metric (QEM), we perform greedy decimation while agglomerating global information about approximation error. In lieu of extrinsic quadrics, however, we store intrinsic tangent vectors that track how far curvature
Hsueh-Ti Derek Liu, Mark Gillespie, Benjamin Chislett, Nicholas Sharp, Alec Jacobson, Keenan Crane (2023). Surface Simplification Using Intrinsic Error Metrics. ACM Trans. Graph., 42(4).
@article{ intrinsic-coarsening,
author = {Hsueh-Ti Derek Liu and Mark Gillespie and Benjamin Chislett and Nicholas Sharp and Alec Jacobson and Keenan Crane},
title = {Surface Simplification Using Intrinsic Error Metrics},
journal = {ACM Trans. Graph.},
issue_date = {August 2023},
volume = {42},
number = {4},
month = jul,
year = {2023},
url = {https://doi.org/10.1145/3592403},
doi = {10.1145/3592403},
publisher = {ACM},
address = {New York, NY, USA},
}

